Large Deviations in Boundary-valued Problems for One-dimensional Markov Chains


Borovkov, Alexandr (Novosibirsk, Russia)
borovkov@math.nsc.ru

Let 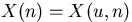$X(n)=X(u,n)$, $n=0,1,\ldots$ -- be a homogeneous in time ergodic Markov Chain on the real line.

The main goal of the paper is to study large deviations problems on asymptotics of

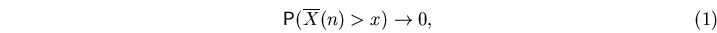
\begin{equation} \mathsf P(\overline{X}(n)\gt x)\to 0,\end{equation}

where

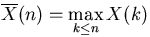$\overline{X}(n)=\mathop{\max}\limits_{k\leq n}X(k)$,

and a more general boundary-valued problem on asymptotics of

\begin{equation} \mathsf P(\max_{k\leq n}\big(X(k)-g(k))\gt\big)\end{equation}

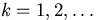for a given arbitrary sequence $g(k)$, $k=1,2,\ldots$.